Remember that agonizing feeling of staring at a geometry problem, the lines and angles blurring together in a dizzying vortex of confusion? I do. It wasn’t until I truly grasped the beauty and logic of right triangles and trigonometry that those anxieties melted away. Unit 8, right triangles and trigonometry, was a turning point in my understanding of math, and it can be for you too! This homework set isn’t just about solving equations; it’s about unlocking a whole new realm of problem-solving and applying it to real-world scenarios. Let’s dive in!

Image: brainly.com
Unveiling the Secrets of Right Triangles
Right triangles, with their one perfectly right angle, are more than just geometric shapes. They’re the foundation of trigonometry, a branch of mathematics that deals with the relationships between the angles and sides of triangles. This unit is all about exploring how the ratios between sides, called trigonometric ratios, can help us solve for unknown angles and sides, opening up a world of possibilities for understanding and measuring the world around us.
Imagine you’re standing on a hill, gazing at a distant landmark. You know the hill’s height and the angle at which you’re looking, but how do you calculate the distance to the landmark? Trigonometry provides the key! Using the trigonometric ratios – sine (sin), cosine (cos), and tangent (tan) – you can establish a link between the angles and sides of the right triangle formed by your position, the landmark, and the ground. This unit will equip you with the tools to master these ratios and apply them to real-world situations.
Delving Deeper into the World of Trigonometry
Understanding the Sine, Cosine, and Tangent
At the core of trigonometry lie the three primary trigonometric ratios: sine, cosine, and tangent. These ratios relate the lengths of the sides of a right triangle to its angles. Let’s break them down:
- Sine (sin): The ratio of the length of the side opposite the angle to the length of the hypotenuse (the longest side).
- Cosine (cos): The ratio of the length of the side adjacent (next to) the angle to the length of the hypotenuse.
- Tangent (tan): The ratio of the length of the side opposite the angle to the length of the side adjacent to the angle.
These ratios are fundamental to solving a vast range of problems. Whether you’re trying to determine the height of a building, the distance to a distant object, or the angles of a complex structure, trigonometry provides the tools to do so. This unit will guide you through mastering these ratios and their applications.
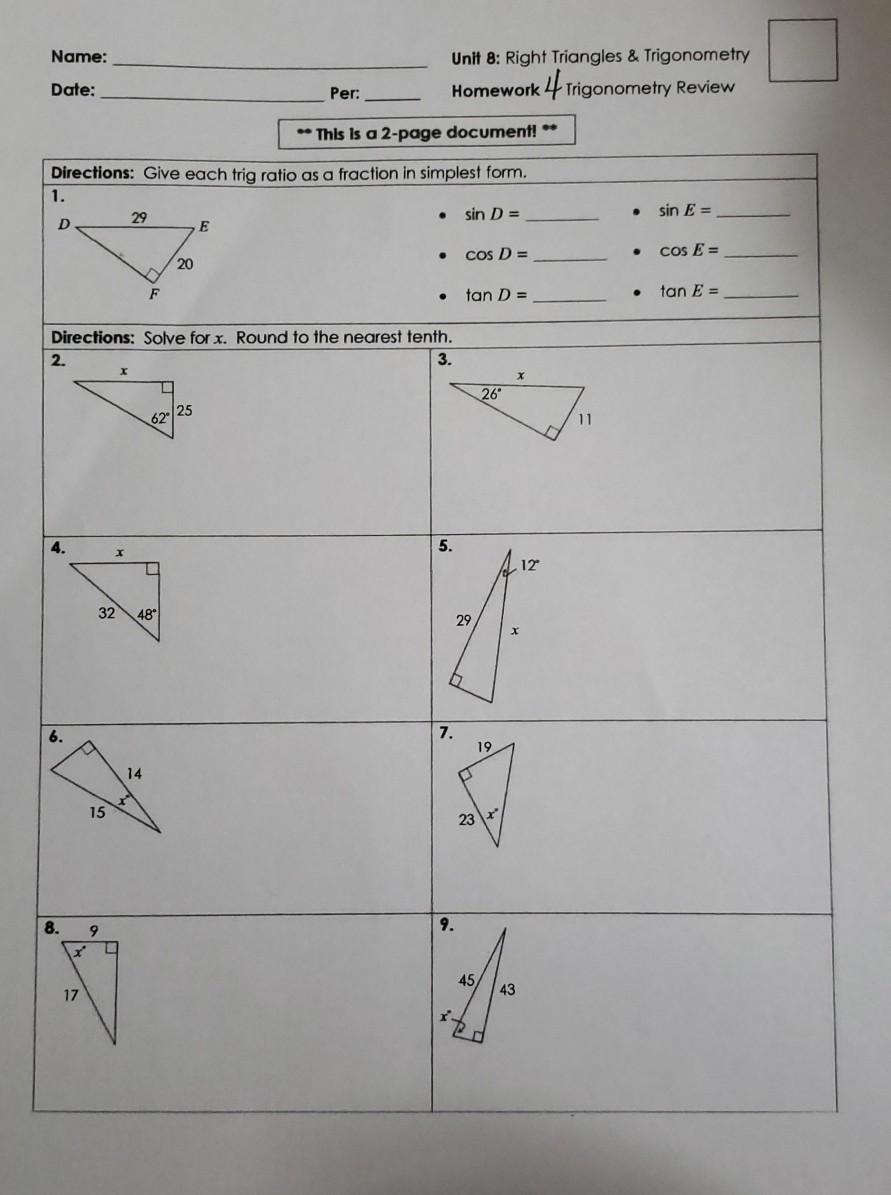
Image: www.chegg.com
The Power of the Pythagorean Theorem
Another vital concept in this unit is the Pythagorean Theorem, a timeless theorem that describes the relationship between the lengths of the sides of a right triangle. It states that the square of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the other two sides. The formula is represented as a² + b² = c², where ‘a’ and ‘b’ are the lengths of the legs, and ‘c’ is the length of the hypotenuse.
The Pythagorean Theorem empowers us to solve for any missing side when two sides are known, making it a cornerstone of right triangle problem-solving. It’s a fundamental tool that you’ll use frequently throughout this unit, and beyond, in various mathematical fields.
Solving for Unknown Angles and Sides
Unit 8 focuses on applying these principles to solve real-world problems. You’ll tackle situations where you need to find unknown angles or sides of right triangles. For instance: Suppose you need to determine the length of a ladder needed to reach a specific height on a wall. Using trigonometry and the Pythagorean Theorem, you can calculate the required ladder length based on the height and the angle formed by the ladder against the wall.
This unit will equip you with the skills and strategies needed to approach these problems confidently. Learning to identify right triangles within larger figures, applying the correct trigonometric ratios, and using the Pythagorean Theorem to find missing side lengths will become second nature.
Applications of Right Triangles and Trigonometry
Right triangles and trigonometry aren’t confined to textbook problems. They have wide-ranging applications in various fields:
- Engineering: Architects and engineers use trigonometry to calculate the angles and lengths of beams, designs, and structures.
- Surveying: Surveyors use trigonometry to measure distances, elevations, and angles in the field.
- Navigation: Pilots and sailors rely on trigonometry for calculating distances and bearings.
- Physics: Trigonometry plays a crucial role in understanding forces, motion, and waves in physics.
By mastering this unit, you’ll gain a deeper understanding of the world around us and how mathematics can be used to solve real-world challenges.
Mastering Unit 8: Right Triangles and Trigonometry Homework 1
Embrace the Basics
Start by solidifying your understanding of the fundamental concepts of right triangles and trigonometry. Review the definitions of sine, cosine, and tangent, and practice calculating them with different triangles. The Pythagorean Theorem is your best friend here—master it, and many problems will fall into place effortlessly.
Visualize and Break Down
When tackling complex problems, visualize the triangle and identify the relevant angles and sides. Break down the problem into smaller steps. Determine what information you have, what you need to find, and the appropriate trigonometric ratio or theorem to use.
Practice Makes Perfect
The key to success in this unit is consistent practice. Work through numerous examples and practice exercises to solidify your understanding. Don’t hesitate to seek help from your teacher, classmates, or online resources when you face difficulties.
FAQ: Right Triangles and Trigonometry
Q1: What is the most challenging part of trigonometry for beginners?
A1: Many beginners find it challenging to memorize and apply the trigonometric ratios correctly. Understanding what each ratio represents and practicing its application will make it much easier.
Q2: Is there a trick to remembering the trigonometric ratios?
A2: Absolutely! A popular mnemonic device is SOH CAH TOA: Sine = Opposite/Hypotenuse, Cosine = Adjacent/Hypotenuse, Tangent = Opposite/Adjacent.
Q3: Where can I find additional help if I’m struggling with this unit?
A3: Your teacher is always a great resource. Additionally, there are excellent online platforms and videos dedicated to explaining trigonometry. Don’t hesitate to explore these resources to find the right approach for you.
Unit 8 Right Triangles And Trigonometry Homework 1
Conclusion
Unit 8, right triangles and trigonometry, is a crucial stepping stone to understanding the world of mathematics. This homework set will equip you with the tools you need to tackle complex challenges. Practice the concepts, visualize the triangles, and remember that trigonometry is a powerful tool for solving real-world problems. Are you ready to conquer the world of right triangles and trigonometry?






