Have you ever wondered how a simple curve on a graph can be translated into a powerful function describing its behavior? Or how a seemingly abstract concept like “limit” can be used to calculate the instantaneous speed of a moving object? This is the magic of calculus, a mathematical tool that unlocks the intricacies of change and motion. At its core lies the field of elementary analysis, which provides the foundation for understanding and applying calculus concepts.

Image: www.enago.com
Elementary analysis, essentially the theory of calculus, forms the bedrock for higher-level mathematical studies, finding applications across diverse fields like physics, engineering, economics, and computer science. In this article, we’ll delve into the fascinating world of elementary analysis, uncovering the essential concepts, techniques, and solutions that make it a cornerstone of modern mathematics.
The Foundations of Elementary Analysis: Building Blocks for Calculus
1. The Language of Numbers: Real Numbers and Their Properties
Elementary analysis begins with the fundamental building blocks of the mathematical world – real numbers. These are the numbers we use everyday, encompassing rational numbers like fractions and decimals, and irrational numbers like pi or the square root of 2. Understanding the properties of real numbers, such as order, completeness, and the concept of limits, forms the groundwork for calculus.
2. Mapping the Terrain: Functions and Their Behavior
Functions are like mathematical blueprints, defining relationships between sets of numbers. In elementary analysis, we explore the behavior of functions, describing how they change, grow, and interact. This involves analyzing key concepts like continuity, differentiability, and integrability, all of which are fundamental to solving calculus problems.
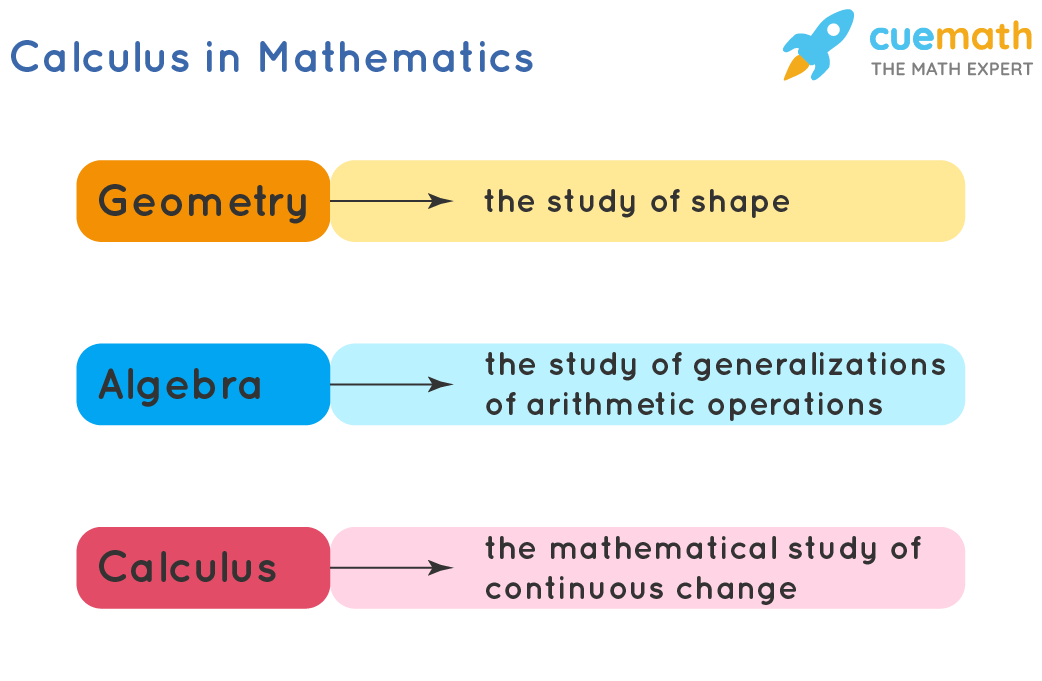
Image: tvsiyengar.com
3. Exploring the Limits: Unraveling the Secrets of Continuity
The idea of a limit is central to the entire field of calculus. It allows us to understand the behavior of a function as its input approaches a particular value. A limit can exist even if the function itself is not defined at that point. This concept is crucial to understanding continuity, which describes the “smoothness” of a function. If a function is continuous, it means we can “draw” its graph without lifting our pen.
Here’s an example: imagine a function describing the temperature of a city throughout the day. If the function is continuous, it means the temperature changes smoothly from one moment to the next. There are no sudden jumps or gaps in the graph. However, in real life, temperatures might have sudden changes due to factors like weather patterns. In those cases, the function wouldn’t be continuous at those specific points.
Navigating the Calculus Landscape: Key Concepts and Techniques
1. The Essence of Change: Derivatives and Rates of Change
The derivative is one of the most powerful concepts in calculus. It represents the instantaneous rate of change of a function at a particular point. Think of a car driving down a road. The speedometer tells you the car’s speed at a specific moment. That speed is the derivative of the function representing the car’s position over time.
2. Unveiling the Area: Integrals and Accumulation
The integral is the counterpart to the derivative. While the derivative tells us about the rate of change, the integral tells us about the accumulation of a function over a certain interval. Imagine a swimming pool being filled with water. The integral of the rate at which water flows into the pool gives us the total amount of water in the pool at a given time.
3. Finding Solutions: Solving Differential Equations
The interplay between derivatives and integrals leads to a powerful tool: differential equations. These equations involve derivatives and functions, and solving them allows us to model and understand a wide range of real-world phenomena. They can be used to describe everything from the growth of populations to the motion of planets around the sun.
For instance, imagine a population of bacteria growing in a petri dish. We can use a differential equation to model how the population changes over time, taking into account factors like the initial population size and the rate of reproduction. By solving this equation, we can predict how the population will grow in the future.
Applications of Elementary Analysis: Unlocking the Secrets of the Universe
The insights gained from elementary analysis are not confined to mathematical frameworks. They extend beyond the realm of abstract theory to power advancements across diverse disciplines:
1. Engineering Marvels: Designing Bridges, Planes, and Beyond
Engineers rely heavily on calculus to design structures, machines, and systems. Techniques based on derivatives help to calculate stresses and strains, allowing for the creation of safe and sturdy buildings, bridges, and aircraft.
2. Modeling Nature’s Mysteries: Unveiling the Secrets of Physics
Physics, the study of the universe, hinges on calculus. From understanding the motion of planets to describing the behavior of subatomic particles, calculus provides the framework for analyzing and predicting physical phenomena.
3. Optimizing Economies: Understanding Growth and Distribution
Economics uses calculus to model complex systems like markets, economies, and financial markets. Derivatives and integrals help analyze supply and demand, optimize production, and predict economic trends.
4. Unlocking the Power of Data: Revolutionizing Computer Science
Calculus plays a crucial role in computer science, especially in fields like numerical analysis, optimization, and machine learning. Algorithms for image processing, pattern recognition, and data analysis all rely on calculus concepts.
Elementary Analysis The Theory Of Calculus Solutions
The Journey Continues: Exploring Further
Elementary analysis is a vast and ever-evolving field. As we’ve seen, its concepts are fundamental to understanding our world and solving complex problems. This article has only scratched the surface, revealing only a glimpse into its vast applications and potential. The journey to mastering elementary analysis, and unlocking its full power, requires dedication, exploration, and a willingness to embrace the beauty of mathematical logic.
So, continue exploring! Dive deeper into the world of calculus, and discover how this remarkable field can illuminate your understanding of the universe around us. Explore online resources, textbooks, and engaging tutorials to further enhance your knowledge and appreciate the profound impact of elementary analysis on our world.






