Have you ever found yourself staring at a textbook, feeling overwhelmed by a sea of equations and abstract concepts? That’s often the experience with linear algebra, a subject that underpins many branches of mathematics, science, and engineering. Fear not, fellow learner! While Linear Algebra Done Right by Sheldon Axler presents a rigorous and elegant approach to the field, it can also feel like navigating a dense forest. But with the right tools and guidance, even the most perplexing theorems can become clear and intuitive. And that’s where the solutions manual for the 4th edition comes into play, serving as your trusted companion on this journey.
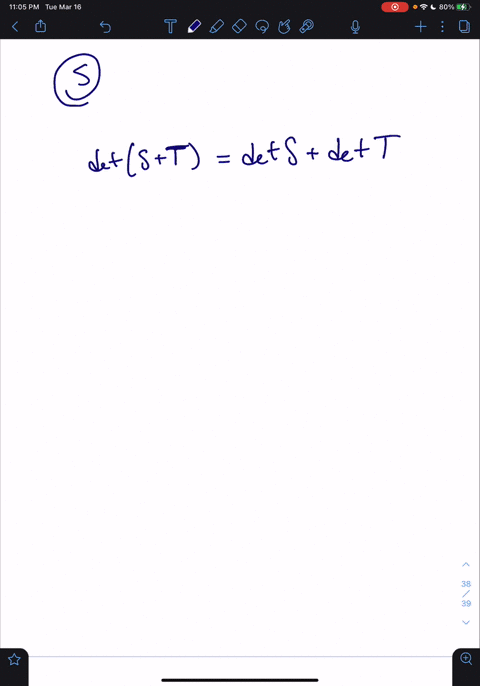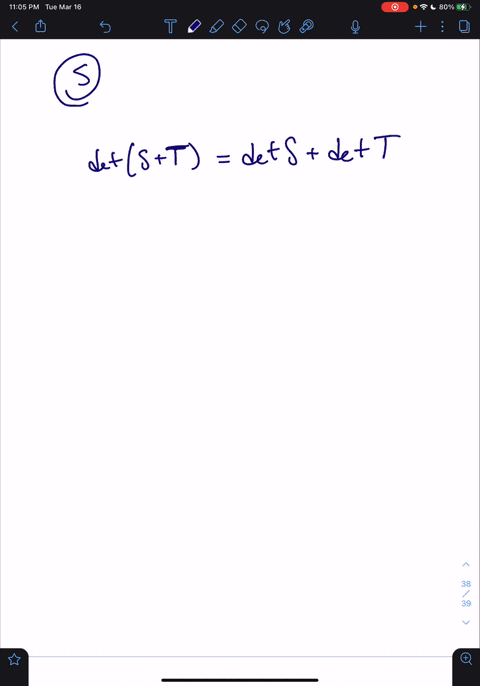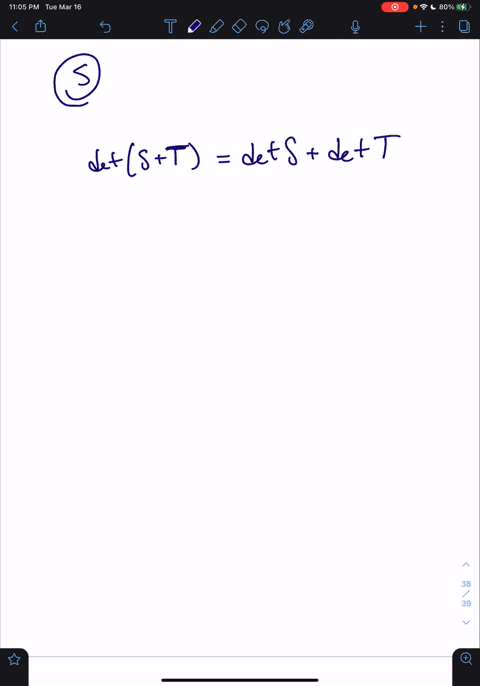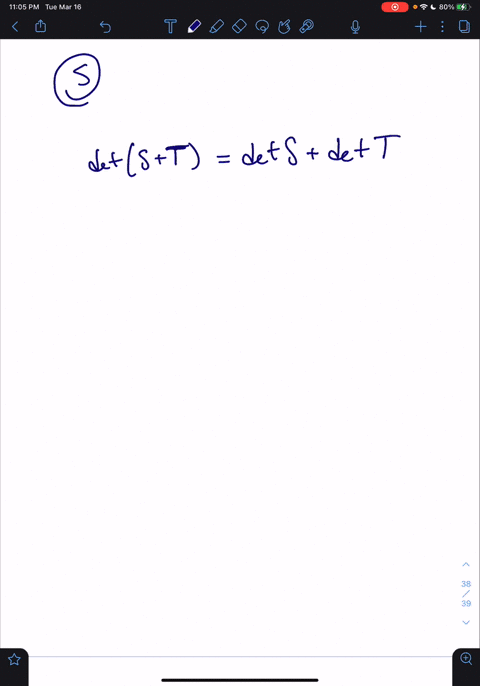
Image: www.numerade.com
This guide isn’t just a collection of answers; it’s a gateway to profound insights and a deeper understanding of linear algebra. Think of it as a virtual mentor, patiently explaining every step, guiding you through the complexities of vector spaces, linear transformations, and eigenvalues. This resource isn’t just for those struggling with homework problems; it’s for anyone who wants to delve deeper into the elegance and power of this fundamental mathematical tool.
Navigating the Labyrinth: Inside the Solutions Manual
The solutions manual for the 4th edition of Linear Algebra Done Right is a treasure trove of illuminating explanations and detailed solutions. It’s a meticulously crafted resource that goes beyond mere answers, offering a comprehensive understanding of the concepts. Each solution is presented in a clear and concise manner, with every step meticulously detailed, making it easier to follow the logic and grasp the underlying principles.
One of the most valuable features of this manual is its focus on building intuitive understanding. Instead of merely providing a string of calculations, it dives into the why behind each step, revealing the elegant connections and relationships within the subject. This approach encourages a deeper engagement with the material, fostering a sense of discovery and true understanding.
For instance, when tackling the complex concept of eigenvalues and eigenvectors, the solutions manual avoids simply plugging numbers into formulas. It instead emphasizes the underlying geometric interpretation of these concepts, showing how eigenvalues and eigenvectors reveal the essential properties of linear transformations, such as scaling, rotation, and reflection.
Beyond the Textbook: The Value of the Solutions Manual
The solutions manual serves as a powerful bridge between the theoretical concepts presented in the textbook and the practical application of linear algebra. It transforms abstract notions into concrete examples, making them relatable and comprehensible. The detailed solutions provide a roadmap for tackling challenging problems, empowering you to develop your problem-solving skills and build confidence in your understanding.
Let’s take a closer look at how the solutions manual can be your trusted companion:
- Mastering the Fundamentals: This resource delves into the core concepts, such as vector spaces, linear transformations, and inner products, offering in-depth explanations and illustrative examples that bring these abstract ideas to life.
- Addressing Challenging Problems: The solutions manual tackles the most challenging problems, providing step-by-step solutions that reveal the intricate connections and logic involved. This empowers you to solve real-world problems that rely on these concepts.
- Building Confidence: The detailed explanations and clear reasoning in the solutions manual boost your confidence in your ability to grasp complex mathematical ideas. It eliminates the ambiguity and uncertainty that can hinder progress in understanding linear algebra.
- Enhancing Your Learning: The manual isn’t just a tool for finding answers, it’s an invaluable learning resource. It encourages active engagement with the material, prompting deeper thought and a greater understanding of the fundamental principles.
Expert Insights: Harnessing the Power of Linear Algebra
Linear algebra is much more than a collection of theorems and equations. It’s a powerful tool that finds applications in a wide range of fields, from computer graphics and machine learning to quantum mechanics and optimization problems. Understanding the principles of linear algebra is essential for those pursuing careers in these areas.
Here are some expert insights that highlight the practical applications of linear algebra:
- Computer Graphics: Linear algebra plays a crucial role in creating realistic images and animations. Transformations like scaling, rotation, and translation rely heavily on linear algebra principles, allowing artists to manipulate objects and create immersive experiences.
- Machine Learning: Many machine learning algorithms rely on linear algebra for tasks such as dimensionality reduction, classification, and regression. The ability to manipulate and analyze data sets using linear algebraic concepts is crucial for building robust machine learning models.
- Optimization: Linear algebra is at the heart of optimization techniques, which aim to find the best solutions for various problems, from resource allocation to financial modeling. Linear programming, a powerful technique for solving optimization problems, is based on linear algebra principles.

Image: quizlet.com
Linear Algebra Done Right Solutions 4th Edition
Embracing the Journey: Unleashing Your Potential
The solutions manual for Linear Algebra Done Right isn’t just a tool; it’s a companion on an exciting journey of intellectual discovery. It empowers you to unravel the complexities of linear algebra, building a solid foundation for future endeavors. Embrace this resource, embrace the challenges, and unlock the boundless potential that lies within this fascinating subject.
This journey goes beyond the classroom, extending into the world of practical applications that shape our modern world. As you delve deeper into the solutions manual, you’ll encounter the elegance and power of linear algebra firsthand, opening doors to a broader understanding of mathematics and its role in shaping our future. And who knows, you might even find yourself applying these concepts in ways you never imagined, contributing to the advancement of technology and innovation.






