Have you ever gazed at a majestic mountain range and wondered how those towering peaks seem to defy gravity, forming such stable triangles? Or perhaps you’ve admired the intricate geometry of a spider’s web, marveling at the perfect angles that define its structure? The world around us is a tapestry of angles, and understanding their properties unlocks a deeper appreciation for the beauty and stability of the universe.

Image: lessonlistshane.z21.web.core.windows.net
Today, we embark on a journey to demystify two fundamental theorems in geometry: the Exterior Angle Theorem and the Triangle Sum Theorem. These powerful principles serve as the cornerstone of understanding angles in triangles and their relationship to each other. Prepare to unlock the secrets hidden within every triangle, as we delve into their intriguing properties and reveal the fascinating interconnectedness of angles.
Unveiling the Exterior Angle Theorem: A Gateway to Unseen Angles
Imagine you’re standing at the vertex of a triangle, looking out at one of its exterior angles. This angle is formed by extending one of the triangle’s sides beyond its vertex. The Exterior Angle Theorem reveals a remarkable truth: this exterior angle is always equal in measure to the sum of the two non-adjacent interior angles. Think of it as a secret code where the exterior angle holds the key to unlocking the values of its hidden internal counterparts.
Let’s visualize this principle. Picture a triangle with angles labeled A, B, and C. Let’s say we’re interested in the exterior angle formed at vertex A when we extend side AB. The Exterior Angle Theorem tells us that the measure of this exterior angle is precisely the sum of the measures of angles B and C. This seemingly simple theorem is the cornerstone for solving many angle-related problems within triangles. It allows us to deduce the measure of a missing angle if we know the measures of its two non-adjacent interior angles, opening a world of possibilities when analyzing geometric figures.
The Triangle Sum Theorem: A Universal Truth
Now, let’s turn our attention to another fundamental principle, the Triangle Sum Theorem. This theorem is perhaps the most famous and widely used in geometry, and it establishes a universal truth: the sum of the interior angles of any triangle always equals 180 degrees. No matter the shape or size of the triangle, the sum of its angles will always add up to 180 degrees. This theorem is as constant as the laws of nature, a testament to the elegant order and predictability woven into the fabric of geometry.
Imagine a bustling city, with each street representing a side of a triangle, and each corner representing its vertex. The Triangle Sum Theorem tells us that no matter how the streets twist and turn, the total sum of the turns at each corner will always be 180 degrees. This theorem is the foundational principle for everything from construction to navigation, ensuring that structures are stable and routes are planned accurately.
Decoding the Interplay: Exterior Angle Theorem + Triangle Sum Theorem
The Exterior Angle Theorem and the Triangle Sum Theorem work hand-in-hand, creating a powerful duo for unraveling the mysteries of angles within triangles. Understanding their relationship unlocks a whole new perspective on geometric problems.
Let’s illustrate this with a simple example. Imagine a triangle where we know the measures of two angles: angle A = 60 degrees and angle B = 80 degrees. We wish to find the measure of the exterior angle formed at vertex A. Here’s where the interplay of the Exterior Angle Theorem and the Triangle Sum Theorem shines.
First, we can use the Triangle Sum Theorem to determine the measure of angle C. Since the sum of the interior angles of a triangle is 180 degrees, we have:
- Angle C = 180 degrees – Angle A – Angle B
- Angle C = 180 degrees – 60 degrees – 80 degrees
- Angle C = 40 degrees
Now, we can leverage the Exterior Angle Theorem to find the measure of the exterior angle at vertex A.
- Exterior Angle at A = Angle B + Angle C
- Exterior Angle at A = 80 degrees + 40 degrees
- Exterior Angle at A = 120 degrees
Therefore, we’ve successfully determined the measure of the exterior angle at A using our knowledge of the Exterior Angle Theorem and the Triangle Sum Theorem, revealing the power of these interconnected principles.
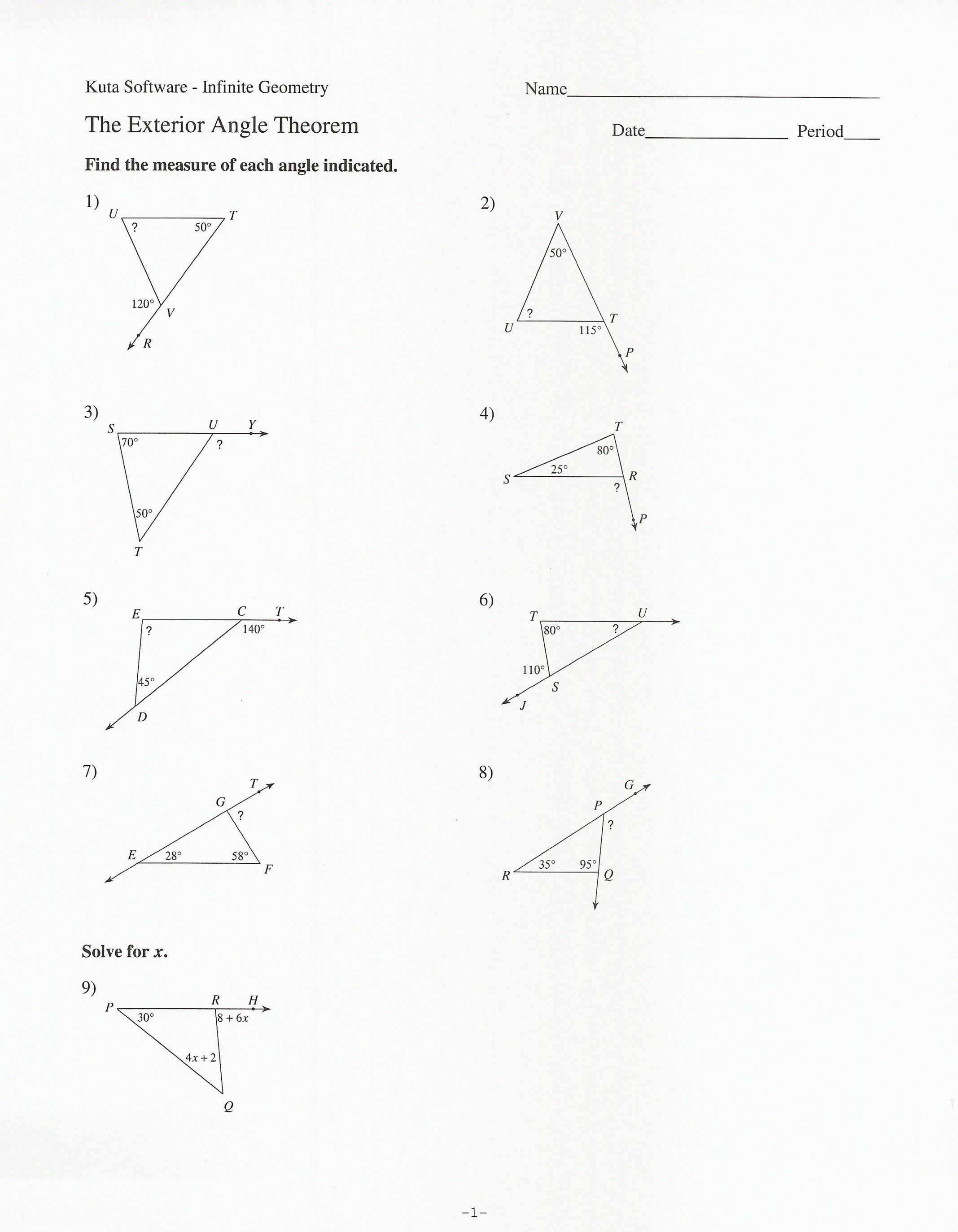
Image: printablelibriglins.z13.web.core.windows.net
The Practical Applications: From Construction to Navigation
These theorems aren’t confined to the abstract realms of geometry; they have far-reaching practical implications, shaping the world around us. Architects rely on these principles to design stable structures, ensuring the stability of bridges, buildings, and even the iconic Eiffel Tower.
Navigation systems rely on the Triangle Sum Theorem to calculate distances and directions, ensuring that airplanes reach their destinations safely and efficiently. Surveyors leverage the power of these theorems to accurately map terrain, ensuring that infrastructure projects are aligned and precise.
3.5 Exterior Angle Theorem And Triangle Sum Theorem Answer Key
Mastering the Theorems: Your Journey Begins Now
Understanding the Exterior Angle Theorem and the Triangle Sum Theorem is not just about memorizing formulas; it’s about unlocking a key to understanding the fundamental order and stability of our world. Whether you’re exploring the intricacies of geometric shapes or tackling real-world problems in architecture, engineering, or navigation, these theorems provide a foundational understanding of angles and their interconnectedness.
So, go forth and explore the fascinating world of triangles, armed with the knowledge of the Exterior Angle Theorem and the Triangle Sum Theorem. Let these powerful principles guide you as you unravel the mysteries of angles, their properties, and their impact on our daily lives. The journey of geometric discovery awaits!






