Have you ever stared at a math problem, feeling overwhelmed by the intricate steps and confusing variables? It’s a common experience, but fear not! Today, we’re going to delve into the fascinating world of substitution, specifically in the context of 6.2 practice problems. This journey will equip you with the knowledge and confidence to tackle these problems with ease.

Image: worksheetmagicritter101.z21.web.core.windows.net
Substitution, at its core, is a powerful tool in algebra. Picture it as a magic trick where you replace complex expressions with simpler ones, making the entire equation more manageable. In 6.2 practice, we often encounter situations where we are given a system of equations, and our goal is to find the values of unknown variables. This is where substitution shines, allowing us to solve these equations efficiently and accurately.
Understanding the Basics: Substitution in a Nutshell
Before diving into the specifics, let’s lay a solid foundation. Substitution is all about replacing a variable with its equivalent value. Imagine you have two equations:
- x + y = 5
- x = y + 2
The beauty of substitution lies in recognizing that we can use the second equation to replace ‘x’ in the first equation. Since we know ‘x’ is equal to ‘y + 2’, we substitute it into the first equation, resulting in:
(y + 2) + y = 5
Now, we have an equation with just one variable, ‘y’. This simplification makes solving for ‘y’ a breeze. Once we know ‘y’, we can substitute its value back into either of the original equations to find ‘x’.
The 6.2 Practice Conundrum: Diving Deeper
In 6.2 practice, we often encounter scenarios involving systems of equations that are more complex than our simple example. We might be dealing with quadratic equations, inequalities, or even systems with three or more variables. While the core concept of substitution remains the same, the execution can become more intricate.
Think of these 6.2 practice problems as puzzles. Each equation presents a piece of the puzzle, and our goal is to find the missing pieces by strategically substituting values. It’s like building a bridge, where each substitution acts as a connecting beam, bringing us closer to the solution.
Mastering the Art: Strategies for Substitution Success
Now, let’s uncover a few key strategies that will empower you to conquer 6.2 practice substitution problems.
- Identifying the Target: The first step is to identify the variable you want to isolate and substitute. Look for an equation where you can easily solve for one variable in terms of the other. In our example, the second equation (x = y + 2) allowed us to isolate ‘x’ directly.
- Strategic Substitution: Once you have the isolated variable, carefully plug its value into the other equation. This will create a new equation with a single variable.
- Solving for the Unknown: Solve the new equation to find the value of the variable you substituted for. This will give you one piece of the puzzle.
- Back Substitution: Substitute the value you just found back into any of the original equations to find the value of the other variable.
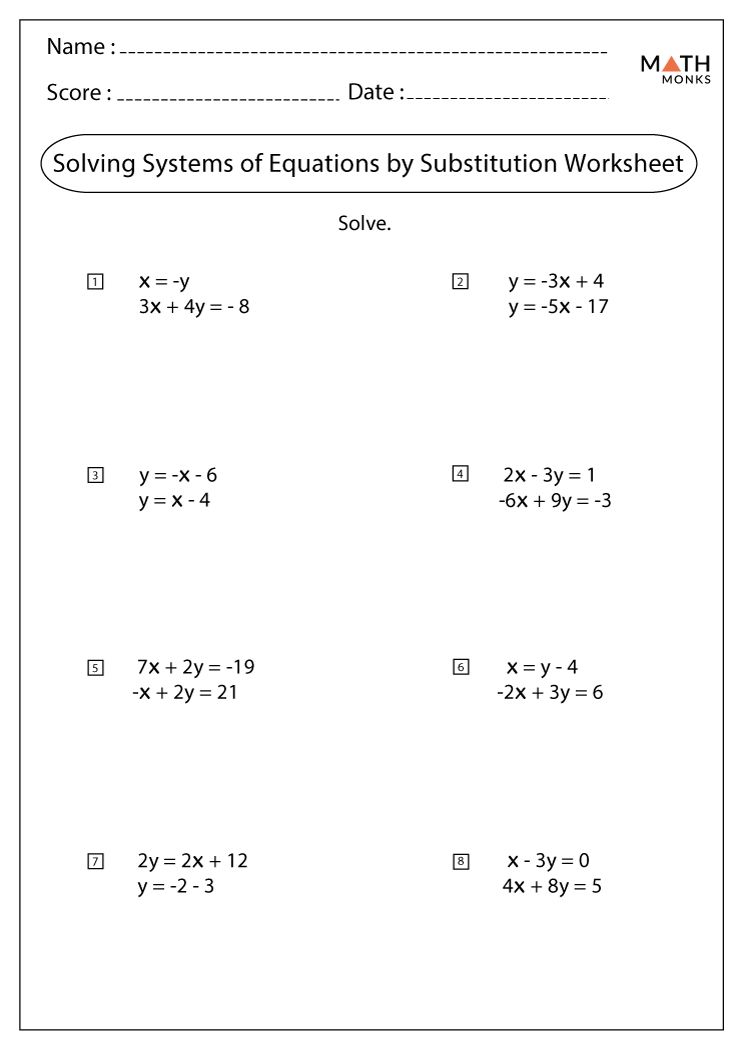
Image: mungfali.com
Expert Advice: Tips from the Trenches
Let’s hear from the experts! Experienced mathematicians often emphasize the importance of careful organization and clear steps. They advise students to write out each step meticulously to avoid errors and ensure a smooth process. They also recommend practicing with various problem types to build confidence and proficiency.
Building Confidence: Practice Makes Perfect
The key to conquering any mathematical concept, especially 6.2 practice substitution, lies in consistent practice. Don’t be afraid to tackle problems of varying difficulty and seek help when needed. The more you practice, the more comfortable and confident you’ll become. Remember, every problem you solve is a step towards mastery.
6 2 Practice Substitution Answer Key With Work
Final Thoughts: Embracing the Journey
As you delve deeper into 6.2 practice substitution, remember that it is not about rushing to find the answer but about embracing the journey of understanding the concepts and developing problem-solving skills. The confidence and knowledge you gain will be invaluable, not just for 6.2 practice, but for your mathematical journey as a whole. So, embark on this exciting adventure with an open mind and a thirst for knowledge, and watch as the world of substitution unfolds before you.






